Articles — Math

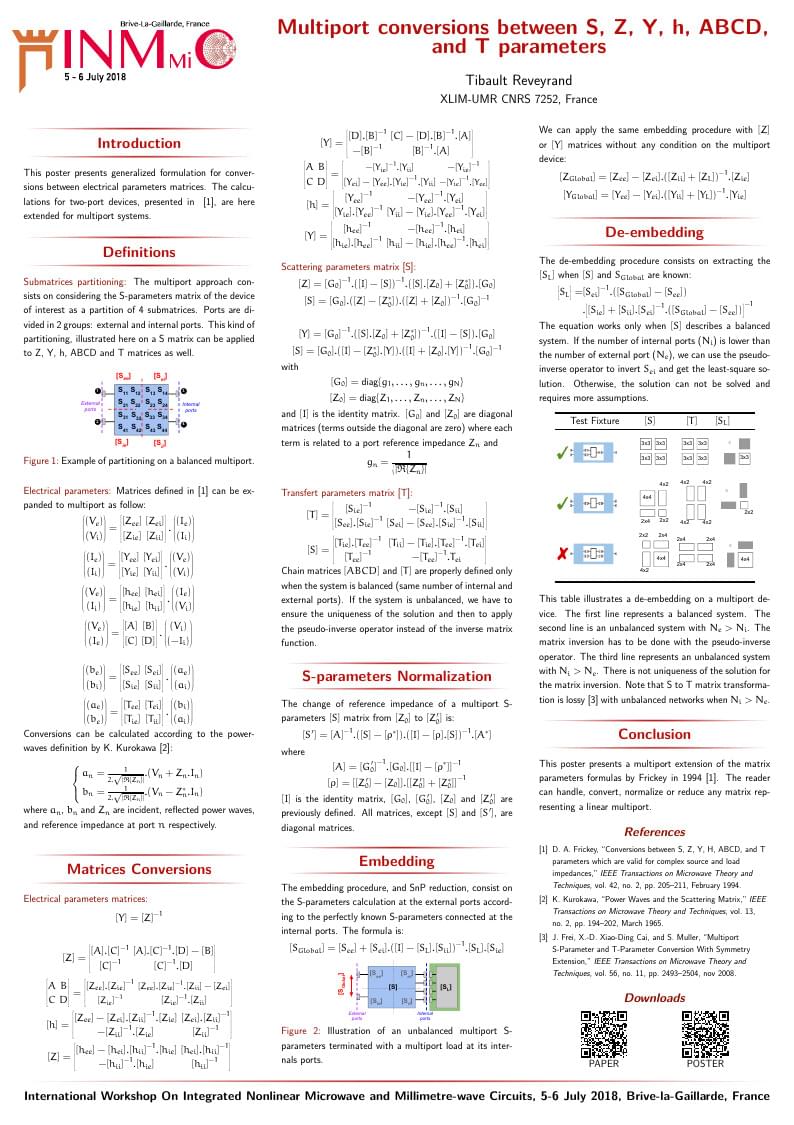
«Multiport conversions between S, Z, Y, h, ABCD, and T parameters.» Integrated Nonlinear Microwave and Millimetre-wave Circuits (INMMIC 2018), Brive-la-gaillarde, France, July 2018. Article: http://www.microwave.fr/publications/151.pdf Poster: http://www.microwave.fr/publications/151p.pdf

Algebra Linear

My documentation report Objetive: Keep track of the notes taken in convex analysis course.

The aim of this laboratory work is to design a strut/bracket assembly for aircrafts. Experiments are carried out to determine mechanical properties of certain materials.The material chosen is Mild Steel. Given the possible condition experienced by the material and the safety factor, the dimensions for the designs of the strut/bracket assembly for aircrafts are obtained to avoid failure by yield or fracture. The diameter of the pin, d ,which is subjected to shear stress should be larger than 14.56mm. The diameter of the rod, D, should be larger than 12.74mm. The thickness of the rod would be 10mm.

In mathematics, a rational number is any number that can be expressed as the quotient or fraction p/q of two integers, a numerator p and a non-zero denominator q. Since q may be equal to 1, every integer is a rational number. The set of all rational numbers, often referred to as ”the rationals”, is usually denoted by a boldface Q (or blackboard bold , Unicode ); it was thus denoted in 1895 by Giuseppe Peano after quoziente, Italian for ”quotient”. The decimal expansion of a rational number always either terminates after a finite number of digits or begins to repeat the same finite sequence of digits over and over. Moreover, any repeating or terminating decimal represents a rational number. These statements hold true not just for base 10, but also for any other integer base (e.g. binary, hexadecimal). A real number that is not rational is called irrational. Irrational numbers include √2, , e, and . The decimal expansion of an irrational number continues without repeating. Since the set of rational numbers is countable, and the set of real numbers is uncountable, almost allreal numbers are irrational.

Estudo de um sistema dinâmico.

Voor seminarie kregen wij de opdracht een moirépatroon op bestelling te maken. We moesten aanvankelijk het niveaulijnpatroon vinden waarvan de glanskrommen afgeronde vierkanten voorstellen. Gezien we hier vrij snel in geslaagd waren, hebben we de opdracht uitgebreid. Ons uiteindelijke doel werd het maken van vier moirépatronen, met name de vier symbolen van het kaartspel. In dit verslag staat stap voor stap uitgeschreven hoe we tot dit resultaat zijn gekomen, van functies met twee variabelen tot het uiteindelijke plotten van de moirépatronen met het computeralgebrapakket Sage.

Cet article étudie deux méthodes utilisées dans le cadre du transport humanitaire en cas de crise (désastre, épidémie...). Le Covering Tour Problem se focalise sur l'équité de distribution des vivres, alors que le Capacitated Vehicle Routing Problem se concentre sur l'urgence de la distribution. Nous proposons une nouvelle approche mélangeant ces deux approches pour former une solution à la fois équitable et rapide. Ce article a été rédigé dans le cadre du TER 2014-2015.

Le modèle linéaire mixte a été mis en oeuvre dès les années 1950, essentiellement dans le domaine de la génétique animale (réf. Henderson[1],[2]). Il n’a toutefois connu une utilisation plus générale qu’au cours des années 1990, en relation avec le développement de nouvelles procédures de calcul dans le cadre des logiciels statistiques. L’utilisation du modèle linéaire mixte soulève, par rapport aux modèles classiques d’analyse de la variance, un certain nombre de difficultés particulières, tant en ce qui concerne l’estimation des différents paramètres que la réalisation des tests d’hypothèses. Des informations peuvent être trouvées à ce sujet dans les articles de Littell [2002], McLean et al. [1991], et Piepho et al. [2003], et dans les livres de Demidenko [2004], McCulloch et Searle [2001],